A close look at quadratic equations

1.    Introduction

A rather common topic in maths: how to best solve quadratic equations. Not really rocket science, but there are a few tricks to do it fast, systematically and minimize chances of errors.

CHOOSE THE SIMPLE METHODS FIRST TO SAVE TIME, DEPENDING ON THE PROBLEM AT HAND !

2.    Sequential approach

Let us take the usual formula for a quadratic equation: f(x) = ax² + bx + c = 0.

1- First, if you can recognize one of the remarkable identities, for instance α² - β² = (α – β) (α + β), then you are immediately done.  For the α² - β² situation, there are two roots corresponding to α = β and α = -β.

 

Example:  f(x) = 4x² – 4x + 1  

This is the same pattern as the identity α² - 2 α β + β² = (α – β)²

Therefore:  f(x) = (2x – 1)² with a double root x = 1/2

 

2- When case 1 is not applicable, maybe it is possible to find an obvious root of the equation by direct observation. This often happens, for instance roots such as 1, 2, -1 ,… Let us call this obvious root x₁ . Since x₁ is a root then you know that f(x) needs to be of the form (x – x₁)(ax +d) and the problem is almost solved. The constant terms in  ax² + bx + c   and     (x – x₁)(ax +d) have to match, therefore c = - d x₁  and d = -c / x₁. As a consequence the second root is x₂ = -d/a = -c/(ax₁)

 

Example:  g(x) = 2x² +5x - 7 = 0   

One obvious solution x = 1

g(x) = (x – 1) (2x + constant); the constant can be easily seen to be equal to 7.

Therefore:  g(x) = (x – 1) (2x + 7) with roots 1 and -7/2

 

3- If the quick first and second methods are not applicable, the traditional “quadratic formula” method including the calculation of the discriminant b² – 4ac can be applied; it works all the time, however at the expense of the required calculations.

 

An interesting and effective alternative to the quadratic formula is to transform f(x) into its so-called “canonical form” a(x- α)² + β . The method is also called “solving by completing the square”:

 

• For the x-terms to match between ax² + bx + c and a(x- α)² + β = a(x² -2αx+α²) + β, you need b = - 2 αa, therefore   α = -b/2a  .

 

• Since ax² is replaced by a(x- α)² in the expression ax² + bx +c, in order to get the constant terms correct, we need to subtract aα²:

f(x) = ax² + bx + c = a(x- α)² - aα² +c ; this gives   β = - aα² + c .  

 

• Conclusion: the canonical form of ax² + bx + c is a(x- α)² + β, with α = -b/2a and β = - aα² +c

.

• The roots can be derived directly from the canonical form by solving a(x- α)² + β = 0, which has real roots when β/a ≤ 0.

 

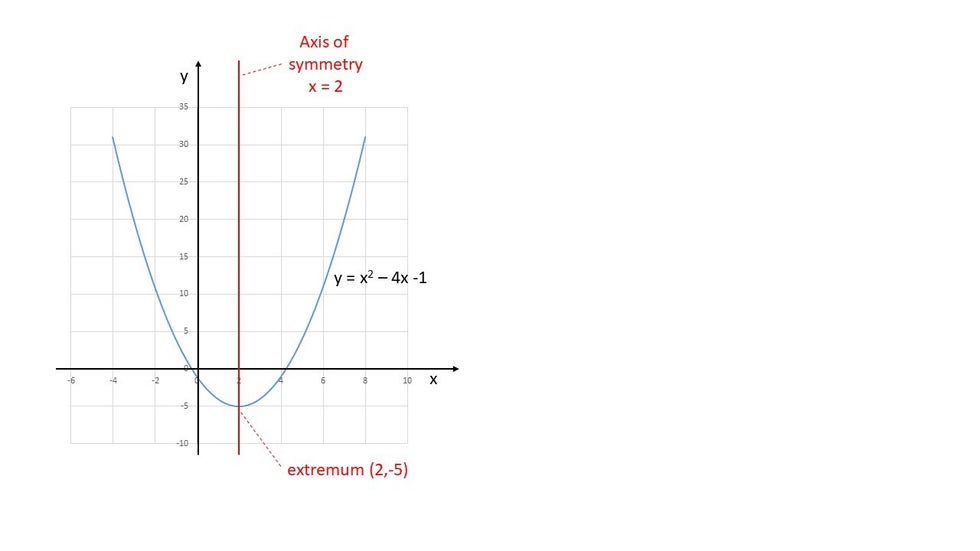
Example:  h(x) = x² – 4x -1   

h(x) can be re-written as h(x) = (x² – 4x + 4) - 4 -1   = (x – 2)² – 5

The roots are such that (x – 2)² = 5     i.e.    x - 2 = ±√5

therefore the two roots are: 2 + √5 ; 2 - √5

 

As a bonus, the canonical form gives directly the axis of symmetry (x = α) of the parabolic curve corresponding to f(x) = ax² + bx + c as well as the coordinates of the extremum (α, β) of the parabol.